Given the next quadratic function:
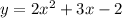
to sketch its graph, first, we need to find its vertex. The x-coordinate of the vertex is found as follows:
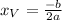
where a and b are the first two coefficients of the quadratic function. Substituting with a = 2 and b = 3, we get:
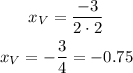
The y-coordinate of the vertex is found by substituting the x-coordinate in the quadratic function, as follows:
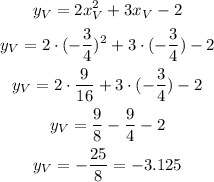
The factorization indicates that the curve crosses the x-axis at the points (-2, 0) and (1/2, 0). We also know that the curve crosses the y-axis at (0,-2). Connecting these points and the vertex (-0.75, -3.125) with a U-shaped curve, we get: