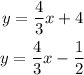Given the equation;
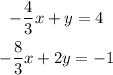
The slope-intercept form is given as;

Rewriting the equations in slope-intercept form gives;

Also;
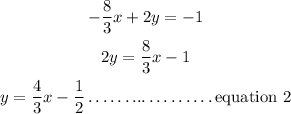
Equation 1 and equation 2 have an equal solution. Thus, the lines are parallel to each other. In otherwords, the lines cannot intersect.
The graph is;
The equations in slope-interceot form are;