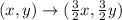By definition, Dilations are transformations in which the Image (the figure obtained after the transformation) and the Pre-Image (the original figure), have different sizes, but their shapes are the same.
When the image is larger than the Pre-Image, the scale factor is greater than 1, and when the Image is smaller than the Pre-Image, the scale factor is between 0 and 1.
Therefore, the scale factor is used to change the sizes of the figures.
In this case, you know that the Pre-Image is the triangle ABC, and its Image is the triangle A'B'C'.
Knowing this vertex of the Pre-Image:

And this vertex of the Image:
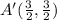
You can identify that A' was obtained by multiplying each coordinate of A by the following scale factor:
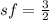
Therefore, you can determine that the Rule for this transformation is:
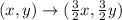
Then, the answer is: