Question

Which equation represents a circle that contains the point (-5, 3) and has a center at (-2, 1)? Distance formula: vaa -02 (x - 1)2 + ( + 2)2 = 25 (x + 2)2 + (-1)=5 O (* + 2) + (-1) - 25 (x-1) + ( + 2) = 5

​

Answer 1

Given:

The center of the circle = (-2,1).

Circle passes through the point (-5,3).

To find:

The equation of the circle.

Solution:

Radius is the distance between the center of the circle and any point on the circle. So, radius of the circle is the distance between the points (-2,1) and (-5,3).

`Distance=√((x_2-x_1)^2+(y_2-y_1)^2)`

`r=√((-5-(-2))^2+(3-1)^2)`

`r=√((-5+2)^2+(2)^2)`

`r=√((-3)^2+(2)^2)`

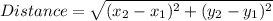
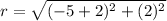
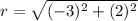
On further simplification, we get

`r=√(9+4)`

`r=√(13)`

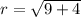
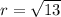
The standard form of a circle is:

`(x-h)^2+(y-k)^2=r^2`

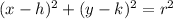
Where, (h,k) is the center of the circle and r is the radius of the circle.

Substitute h=-2, k=1 and
`r=√(13)`.

`(x-(-2))^2+(y-1)^2=(√(13))^2`

`(x+2)^2+(y-1)^2=13`

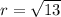Therefore, the equation of the circle is
`(x+2)^2+(y-1)^2=13`.