The sople of two perpendicular lines are opposite reciprocals. So if you have the slope of one line and need to know the slope of the line perpendicular to it you need to know that:

Where
m1: the slope of the first line
m2: the slope of the second line
In this case
m1= ?
m2=5
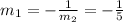 The slope of the line perpendicular to y=5x+2 is -1/5
The slope of the line perpendicular to y=5x+2 is -1/5To determine the y intercept we have to find first the equation of the line:

We have the m = -1/5
The b is calculated using the point (-4 , -7), as follow:
x=-4
y=-7

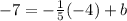
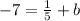

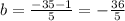
The equation of the line is:

Knowing this we determine the y intercept when x=0:
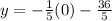

Then, the y intercept is : -36/5