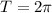Given: A trigonometric function
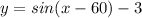
Required: To find out the amplitude, period, vertical shift, and phase shift.
Explanation: The trigonometric function of the form
![y=Asin[B(x+C)]+D\text{ ........\lparen1\rparen}](https://img.qammunity.org/2023/formulas/mathematics/college/ml3spqpcwpb3c7c2i0ho9mhyyz8smeqo03.png)
has

If C is positive shift is towards the left, if C is negative shift is toward the right.
Similarly, if D is positive shift is upwards otherwise for negative D shift is downwards. Now comparing the given function we get the following values
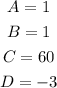
Hence amplitude A=1. Now for the time period T
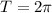
The phase shift is C=-60, and the vertical shift is D=-3.
Final Answer: Amplitude=1
Phase shift=-60
Vertical shift=-3
Time period is-