We have to solve the expression:
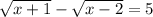
We can start by finding the domain of possible values of x.
The argument of the square root can not be negative, so we can limit the values of x as:
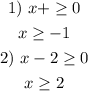
Then, x has to be greater than 2, as this interval is more restrictive.
When x gets very large, the difference between the two roots approaches 0.
Also, the difference of this square roots decreases with the increase of x, so the maximum value happens for x = 2, which is the minimum value of x in the domain.
We then can calculate the value for the difference of square roots when x = 2 as:
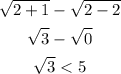
Then, one side of the equation has a maximum value that is the square root of 3. This maximum value is already smaller than 5, so there is no value of x that can make this expression equal to 5.
Answer: there is no solution for x.