Question

Emily, with a mass of 50.0 kilograms, is riding on a wagon of mass 14.2 kilograms with a velocity of +4.27 meters per second. She then jumps off the wagon and lands on the ground with a horizontal velocity of +5.41 meters per second. What is the velocity of the wagon after Emily jumps off? Include units in your answer.

Answer 1

Given information:

Mass of Emily,

`m_1=50\text{ kg}`

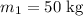
Mass of the wagon;

`m_2=14.2\text{ kg}`

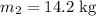
Initial velocity;

`u=4.27\text{ m/s}`

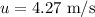
Final velocity of Emily;

`v_1=5.41\text{ m/s}`

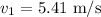
According to the conservation of momentum the initial momentum of the system equals the final momentum of the system.

`(m_1+m_2)u=m_1v_1+m_2v_2`

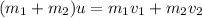
Here, v_2 is the velocity of the wagon after Emily jumps off.

The velocity of the wagon after Emily jumps off is given as,

`\begin{gathered} m_2v_2=(m_1+m_2)u-m_1v_1 \\ v_2=((m_1+m_2)u-m_1v_1)/(m_2) \end{gathered}`

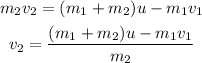
Substituting all known values,

`\begin{gathered} v_2=\frac{\lbrack(50\text{ kg})+(14.2\text{ kg})\rbrack*(4.27\text{ m/s})-(50\text{ kg})*(5.41\text{ m/s})}{(14.2\text{ kg})} \\ \approx0.26\text{ m/s} \end{gathered}`

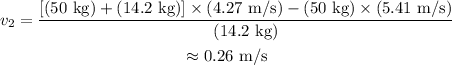
Therefore, the velocity of the wagon after Emily jumps off is 0.26 m/s.