step 1
Find out the radius of the circle
Remember that
the circumference of the circle is
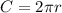
C=6pi units -----> given
substitute
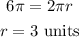
step 2
Find out the area of the complete circle

Remember that the area of the complete circle subtends a central angle of 360 degrees
so
Applying proportion find out the area by a central angle of 72 degrees
9pi/360=x/72
solve for x
x=(9pi/360)*72
x=1.8pi unit2
therefore
The area of the shaded region is 1.8pi unit2
Find out the error