Question

Use the triangle below to solve for the indicated angle.

Answer 1

`\angle x\approx39^(\circ),\angle y\approx51^(\circ)`

1) We were told to find the measure of each angle.

2) So, let's make use of some trig ratios. And let's find the measure of ∠x

`\begin{gathered} \sin (x)=(5)/(8) \\ (x)=\sin ^(-1)((5)/(8)) \\ x=38.68^(\circ) \end{gathered}`

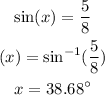
Notice that since we need to find the measure of the angle we had to resort to the arcsine of (5/8) to find that.

So, let's now, find the measure of ∠y

`\begin{gathered} \cos (y)=(5)/(8) \\ y=\cos ^(-1)((5)/(8)) \\ y\approx51.32^(\circ) \end{gathered}`

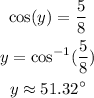
So let's round them off to the nearest whole number. Then the answer is:

`\angle x\approx39^(\circ),\angle y\approx51^(\circ)`