Question

Use the quadratic formula to solve for x. 6x²-2x=1

Answer 1

`\begin{gathered} x_1=0.61 \\ x_2=-0.27 \end{gathered}`

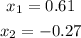
Step-by-step explanation

when you have a quadratic equation in the form:

`ax^2+bx+c=0`

the solution to find x is given by the quadratic formula:

`x=\frac{-b\pm\sqrt[]{b^2-4ac}}{2a}`

then

Step 1

reorder the equation, let the zero in the rigth side

`\begin{gathered} 6x^2-2x=1 \\ \text{subtract 1 on both sides} \\ 6x^2-2x-1=1-1 \\ 6x^2-2x-1=0 \end{gathered}`

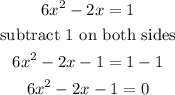
Step 2

identify a, b and c and replace in the formula

`\begin{gathered} 6x^2-2x-1=0\leftrightarrow ax^2+bx+c=0 \\ therefore \\ a=6 \\ b=-2 \\ c=-1 \end{gathered}`

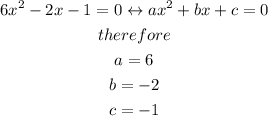
now, replace in the formula.

`\begin{gathered} x=\frac{-b\pm\sqrt[]{b^2-4ac}}{2a} \\ x=\frac{-(-2)\pm\sqrt[]{(-2)^2-4(6)(-1)}}{2(6)} \\ x=\frac{+2\pm\sqrt[]{4+24}}{12} \\ x=\frac{+2\pm\sqrt[]{28}}{12} \\ x=\frac{+2\pm\sqrt[]{7\cdot4}}{12} \\ x=\frac{+2\pm2\sqrt[]{7}}{12} \\ x=\frac{1\pm\sqrt[]{7}}{6} \end{gathered}`

so, the solutions of the equation are

`\begin{gathered} x_1=\frac{1+\sqrt[]{7}}{6}=(1+2.64)/(6)=0.6076\approx0.61 \\ \text{and} \\ x_2=\frac{1-\sqrt[]{7}}{6}=-0.27429\approx-0.27 \end{gathered}`

I hope this helps you