Question

You deposit $ 342 each year into an IRA earning 6.9 % interest compounded annually. How much will you have in the account in 3 years?

Answer 1

Given:

Principal, P = $342

Interest rate, r = 6.9% = 0.069

number of times compounded, n = annually = 1

Time, t = 3 years

Let's find the amount in the account after the given time.

Apply the compound interest formula:

`A=P(1+r)^t`

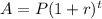
Where:

P = $342

r = 0.069

t = 3

A is the amount after the given time.

Thus, we have:

`\begin{gathered} A=342(1+0.069)^3 \\ \\ A=342(1.069)^3 \\ \\ A=342(1.221611509) \\ \\ A=417.79 \end{gathered}`

Therefore, the amount in the account after 3 years will be $417.79

ANSWER:

$417.79