Let J be how much Jean had initially, I be how much Irene had initially and T be how much Todd had initially.
After Jean gave $10 to Irene, they ende up with:
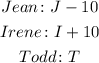
After Irene gave $6 to Tood, they ended up with:

And the question says that, after all this, Jean had $10 more than Irene and 20$ more than Todd, that is:

We want to find out how much more Jean had originally than the others, that is:
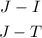
Using the first expression that we got, we can find the first answer:
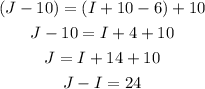
Thus, Jean had $24 more than Irene originally.
Using the second, we can find out the other difference:

Thus, Jean had $36 more than Todd originally.
The alternative that matches the answer is D.