Question

Find all points on the y-axis which are 8 units from (−7, 3).(x, y) = (smaller y-value)(x, y) = (larger y-value)

Answer 1

The distance between two points is given by the following formula:

`d=\sqrt[]{(x_2-x_1)^2+(y_2-y_1)^2}`

The given point (-7,3) represents point number 1 (x1,y1).

There are two possible points on the y-axis which are 8 units from the initial point. As they are on the y-axis, the x-coordinate x2 is equal to 0, replace these values in the distance formula (with a distance equal to 8) and solve for y2:

`\begin{gathered} 8=\sqrt[]{(0-(-7))^2+(y_2-3)^2} \\ 8=\sqrt[]{(7)^2+(y_2-3)^2} \\ 8=\sqrt[]{49+(y_2-3)^2} \\ \text{Square both sides} \\ 8^2=\sqrt[]{49+(y_2-3)^2}^2 \\ 64=49+(y_2-3)^2 \\ 64-49=(y_2-3)^2 \\ 15=(y_2-3)^2 \end{gathered}`

Now, let's apply the formula for the square of a difference:

`\begin{gathered} 15=y^2_2-2\cdot y_2\cdot3+3^2 \\ 15=y^2_2-6y_2+9^{} \\ 0=y^2_2-6y_2+9-15 \\ 0=y^2_2-6y_2-6 \end{gathered}`

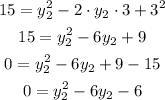
Apply the quadratic formula to solve for y2:

`\begin{gathered} y_2=\frac{-b\pm\sqrt[]{b^2-4ac}}{2a} \\ \text{where a=1, b=-6 and c=-6} \\ y_2=\frac{-(-6)\pm\sqrt[]{(-6)^2-4(1)(-6)}}{2(1)} \\ y_2=\frac{6\pm\sqrt[]{36+24}}{2} \\ y_2=\frac{6\pm\sqrt[]{60}}{2} \\ y_2=(6\pm7.75)/(2) \\ \text{Then } \\ y_2=(6+7.75)/(2)=6.873\text{ and }y_2=(6-7.75)/(2)=-0.873 \end{gathered}`

Thus, the two points which are 8 units from (-7,3) are:

(0,-0.873) smaller y-value and (0,6.873) larger y-value.