Since the production of y must exceed the production of x by at least 100 units, we can write a second inequality:

Looking at the function P, we can see that the coefficient multiplying y is greater than the coefficient multiplying x, therefore increasing y instead of x will have a bigger increase in P.
But from the inequality x + 2y <= 1400, we can see that the "cost" of producing y is two times the "cost" of producing x, that is, for one y produced, we could have produced 2x instead.
The coefficient of y is greater, but it's not more than 2 times greater, therefore it's better to produce x than y.
Since y needs to be at least 100 more than x, let's choose the minimum amount of y to satisfy the inequalities:
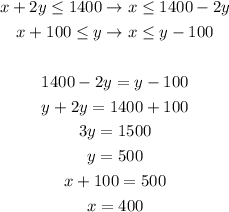
Therefore the values of x and y that give the maximum profit are x = 400 and y = 500.
Graphing the two inequalities, we have:
The feasible region is the intersection region (between red and blue).
The vertices are:
(0, 100), (400, 500), (0, 700).
Calculating the maximum profit (with vertex (400, 500)), we have:
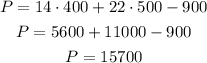
Therefore the production that yields the maximum profit is x = 400 and y = 500, and the maximum profit is P = 15700.