Question

Then, give the vertex of its graph.Write the quadratic function in the form g(x) = a * (x - h) ^ 2 + k g(x) = - 3x ^ 2 - 12x - 8

Answer 1

Given:

Function is

`g(x)=-3x^2-12x-8`

To find:

Write function in the form

`g(x)=a(x-h)^2+k`

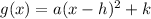
Then give its vertex.

Step-by-step explanation:

We will try to make perfect square of terms.

vertex of

`=ax^2+bx+c\text{ is }(-b)/(2a)`

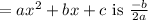
Solution:

We will solve equation by first making perfect square of terms as:

`\begin{gathered} g(x)=-3x^2-12x-8 \\ We\text{ will add or subtract 4 on RHS} \\ g(x)=-3x^2-12x-8-4+4 \\ g(x)=-3x^2-12x-12+4 \\ g(x)=-3(x^2+4x+4)+4 \\ g(x)=-3(x+2)^2+4 \end{gathered}`

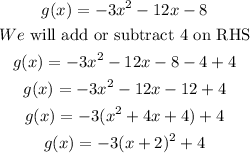
Now, vertex is

`\begin{gathered} h=-(b)/(2a) \\ =-(12)/(6) \\ =-2 \end{gathered}`

Now,

`\begin{gathered} k=f(-2)=(-3*4)-(12*(-2))-8 \\ f(-2)=4 \end{gathered}`

So, vertex is (-2, 4).

Hence , these are the answers.