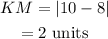Answer:
D. 2 units
Step-by-step explanation:
The coordinates of point K and point M are K(-2,8) and M(-2, 10) respectively.
To find the distance between point K and point M, we use the distance formula:

Therefore:
![\begin{gathered} KM=\sqrt[]{(-2-(-2))^2+(10-8_{})^2} \\ =\sqrt[]{(-2+2)^2+(2_{})^2} \\ =\sqrt[]{0^2+(2_{})^2} \\ =\sqrt[]{4} \\ =2\text{ units} \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/kpkkpcthriq3370z9giad6rw7yxlgzx5s7.png)
The distance between K and M is 2 units.
Alternate Route
Observe that the x-coordinates of K and M are the same. (-2).
Therefore, use the y-coordinate to find the distance between K and M.