Question

18. Craft boxes are sold in containers shaped like a cone or a cylinder. The dimensions are shown in the drawing. Which statement about the volumes of the cylinder and cone is true? A. The volume of the cone is about 2,261.95 cubic centimeters less than the volume of the cylinder. B. The volume of the cylinder is congruent to the volume of the cone. C. The volume of the cone is about 2,261.95 cubic centimeters greater than the volume of the cylinder. D. The volume of the cylinder is about 2,261.95 cubic centimeters less than the volume of the cone.

Answer 1

To answer the question we need to find the volume of each container.

The volume of a cone is given by:

`V=(1)/(3)\pi r^2h`

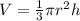
from the figure r=6 and h=12. Plugging this values we have:

`\begin{gathered} V=(1)/(3)\pi(6)^2(12) \\ =144\pi \end{gathered}`

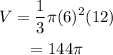
The volume of a cylindir is given by:

`V=\pi r^2h`

plugging the values we have:

`\begin{gathered} V=\pi(12^2)(6) \\ =864\pi \end{gathered}`

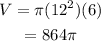
Now, we notice that the volume of the cylinder is bigger than the cone, to find how much we substract them:

`\begin{gathered} 864\pi-144\pi=720\pi \\ \approx2261.95 \end{gathered}`

Therefore we conclude that:

The volume of the cone is about 2,261.95 cubic centimeter less than the volume of the cylinder. (The answer is option A.)