Question

Use the change of base property to rewrite the given expression in terms of natural logarithm or common logarithm

Answer 1

Step-by-step explanation

To rewrite the given logarithm in terms of a common logarithm, apply the following law of logarithm to rewrite it in terms of base 10:

`\log_ab=(\log_(10)b)/(\log_(10)a)`

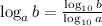
Therefore, the given logarithm becomes:

`(\log_(10)17.3)/(\log_(10)0.2)`

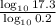
To evaluate the expression, use a calculator or logarithm table to find the value of the logarithms and simplify:

`\begin{gathered} (1.2380)/(-0.6990) \\ -1.7712 \end{gathered}`

That is the answer.