Question

Of each interior angle of a regular hexagon measures(15x - 45)°, find the value of x.

Answer 1

A regular hexagon is a polygon with six sides.

The sum of the interior angles of a polygon is calculated as: (2n-4)90

where 'n' represents the number of sides of the polygon.

from the question provided, each interior angle of the regular hexagon is (15x-45). Therefore, the sum of the interior angles of the regular hexagon will be given by 6(15x-45).

`\begin{gathered} \text{The resulting equation becomes: } \\ 6(15x-45)=(2n-4)90 \\ By\text{ expanding the parenthesis and replacing the value of 'n' to be 6, we have:} \\ 90x-270=\mleft\lbrace2(6\mright)-4\}90 \\ 90x-270=(12-4)90 \\ 90x-270=8(90) \\ 90x-270=720 \\ 90x=720+270 \\ 90x=990 \\ x=(990)/(90) \\ x=11 \end{gathered}`

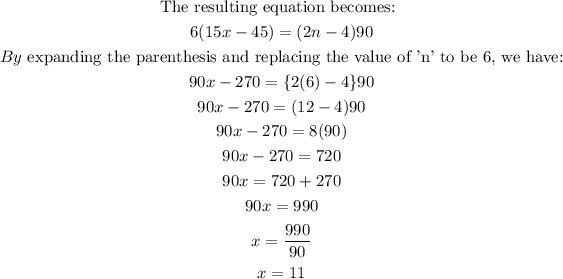
Hence, the value of x is 11