We are given that the graph of the function passes through (-4,19), and (7,3), therefore:
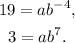
Dividing the second equation by the first one, we get:
![\begin{gathered} (3)/(19)=(ab^7)/(ab^(-4))=(3)/(19)=b^(7+4), \\ b^(11)=(3)/(19), \\ b=\sqrt[11]{(3)/(19)}. \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/gugy2bqbij0ret1psoih5txvvdic44od3p.png)
Now, substituting the above result in the second equation, we get:
![\begin{gathered} a(\sqrt[11]{(3)/(19)})=3, \\ a=\frac{3}{(\sqrt[11]{(3)/(19)})^7}. \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/3teq1yeetygo7gou990gc59yjwcu1hk3px.png)
Rounding b to 4 decimal places, we get:
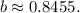
Answer:
![\begin{gathered} a=\frac{3}{(\sqrt[11]{(3)/(19)})^7}, \\ b=0.8455. \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/4n5bpgt0dtm8770tmoanwsvj6ytohx67v7.png)