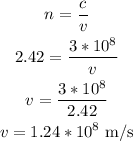Question

6. A light ray travels through the water (n1 =1.33) at theta = 15° until it enters a diamond ring(n2=2.42)a. What is the angle of the refracted light?|b. Given the index of refraction for diamond and the speed of light constant, what isthe velocity of light in diamond?

Answer 1

a.

In order to calculate the angle of refraction, we can use the law of refraction below:

`n_1sin\theta_1=n_2sin\theta_2`

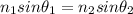
Where n1 and n2 are the index of refraction and theta1 and theta2 are the incident and refracted angles.

So, using n1 = 1.33, theta1 = 15° and n2 = 2.42, we have:

`\begin{gathered} 1.33*sin\left(15°\right)=2.42*sin\theta_2 \\ 1.33*0.258819=2.42*sin\theta_2 \\ 0.34422927=2.42*sin\theta_2 \\ sin\theta_2=(0.34422927)/(2.42) \\ sin\theta_2=0.1422435 \\ \theta_2=8.18° \end{gathered}`

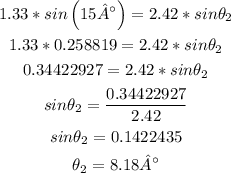
b.

To calculate the velocity of light in diamond, we can use the formula below:

`\begin{gathered} n=(c)/(v) \\ 2.42=(3*10^8)/(v) \\ v=(3*10^8)/(2.42) \\ v=1.24*10^8\text{ m/s} \end{gathered}`