Question

For each of the following pairs of points, find the length of ABa. A(0,2), B(0,1)b. A(0,10), B(24,0)C. A(5,25), B( 16,-

Answer 1

The rule of the distance between the two points (x1, y1) and (x2, y2) is

`d=√((x_2-x_1)^2+(y_2-y_1)^2)`

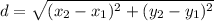
a.

A = (0, 2) and B = (0, 1)

Substitute (x1, y1) by (0, 2) and (x2, y2) by (0, 1) in the given rule above

`\begin{gathered} AB=√((0-0)^2+(1-2)^2) \\ AB=√(0+1) \\ AB=√(1) \\ AB=1 \end{gathered}`

The length of AB is 1

b.

A = (0, 10) and B = (24, 0)

Substitute (x1, y1) by (0, 10) and (x2, y2) by (24, 0)

`\begin{gathered} AB=√((24-0)^2+(0-10)^2) \\ AB=√(576+100) \\ AB=√(676) \\ AB=26 \end{gathered}`

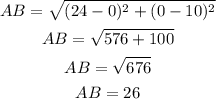
The length of AB is 26

c.

A = (5, 25) and B = (-16, -1)

Substitute (x1, y1) by (5, 25) and (x2, y2) by (-16, -1)

`\begin{gathered} AB=√((-16-5)^2+(-1-25)^2) \\ AB=√((-21)^2+(-26)^2) \\ AB=√(441+676) \\ AB=√(1117) \end{gathered}`

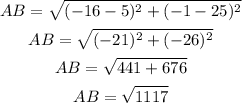
The length of AB is square root 1117