Question

I need help with my math

Answer 1

Question:

Solution:

Let the following linear function:

`4x\text{ + 5y = 20}`

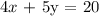
solve for 5y:

`5y\text{ = }20-4x`

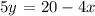
solving for y, we get:

`y\text{ = }-(4)/(5)x\text{ + }4`

the y- intercept is when x = 0, then replacing x = 0 into previous equation we get:

`y\text{ = }-(4)/(5)(0)\text{ + }4\text{ = 4}`

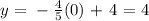
then the y-intercept is the point:

(x,y) = (0,4)

Now, the x-intercept is when y = 0, the replacing y = 0 into the equation:

`y\text{ = }-(4)/(5)x\text{ + }4`

we get:

`0\text{ = }-(4)/(5)x\text{ + }4`

this is equivalent to:

`-4\text{= }-(4)/(5)x\text{ }`

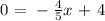
this is equivalent to:

`4\text{= }(4)/(5)x\text{ }`

solving for x, we get:

`x\text{ = }(20)/(4)\text{ = 5}`

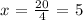
then the x-intercept is the point:

(x,y) = (5,0)

Then, we can conclude that the correct answer is:

1. the y-intercept is the point (x,y) = (0,4).

2. the x-intercept is the point (x,y) = (5,0).

and the graph of the line is: