Question

The graph of f(x) = (1/4)3^x - 6 is shown below. g(x) is a transformation of f(x).How would you write the equation for the function g(x)?

Answer 1

Looking at the graph of g(x) (the red graph), we can see that it has the same format of f(x), but it is 9 units above it (f(x) passes through (0, -6) and g(x) passes through (0, 3)).

Therefore the graph of g(x) can be created using a translation of 9 units up from the graph of f(x).

That means we can write the following expression:

`\begin{gathered} g(x)=f(x)+9\\ \\ g(x)=(1)/(4)3^x-6+9\\ \\ g(x)=(1)/(4)3^x+3 \end{gathered}`

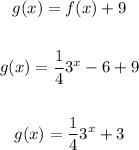
So the correct option is B.