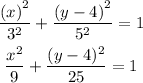Question

Find the equation of the following an ellipse based on the following information: Foci: (0,0), (0,8),Major axis of length 10, and a minor axis of length 6.

Answer 1

We have the next information

Foci: (0,0), (0,8),

Mayor axis =10

Minor axis =6

We will use the next form of the equation of the parabola

`((x-h))/(b^2)+((y-k)^2)/(a^2)`

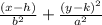
Then we need to know a and b

a=10/2=5

b=6/2=3

and the center is (0,4) in the middle of the points (0,0) and (0,8)

h=0

k=4

The equation of the ellipse is

`\begin{gathered} (\left(x\right)^(2))/(3^(2))+(\left(y-4\right)^(2))/(5^(2))=1 \\ \frac{x^2}{9^{}}+((y-4)^2)/(25)=1 \end{gathered}`