Question

Create a system of equations that includes one linear equation and one quadratic equation. Graph your system of equations and show the solution graphically to verify your solution.

Answer 1

To solve this, we need to propose two equations, one linear, and one quadratic.

We can propose:

`x+y=12`
`y=-2x^2+3x+20`

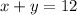
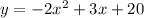
The system is:

`\begin{cases}x+y={12} \\ y={-2x^2+3x+20}\end{cases}`

Now, we need to solve the system of equations. If we solve the first equation for y, then we can substitute in the second equation:

`y=12-x`

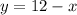
And substitute:

`12-x=-2x^2+3x+20`

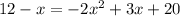
Next, we want to leave a '0' on one side of the equation:

`\begin{gathered} 0=-2x^2+3x+x+20-12 \\ . \\ 0=-2x^2+4x+8 \end{gathered}`

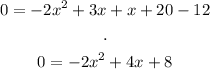
We know how to find the solution to this equation, using the quadratic formula:

`x_(1,2)=(-4\pm√(4^2-4\cdot(-2)\cdot8))/(2\cdot(-2))`

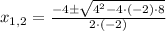
And solve:

`x_(1,2)=(-4\pm√(16+64))/(-4)=(4\pm√(80))/(4)=(4\pm4√(5))/(4)=1\pm√(5)`

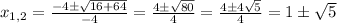
Now, we can find the y-values of the solutions, using the first equation in the system:

`\begin{gathered} (1+√(5))+y=12\Rightarrow y=12-(1+√(5))=11-√(5) \\ . \\ (1-√(5))+y=12\Rightarrow y=12-(1-√(5))=11+√(5) \end{gathered}`

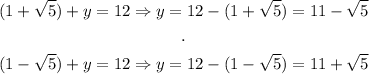
Thus, the two solutions to this system are:

`\begin{gathered} Solution\text{ }1:(1+√(5),11-√(5))\approx(3.236,8.764) \\ Solution\text{ }2:(1-√(5),11+√(5))\approx(-1.236,13,236) \end{gathered}`

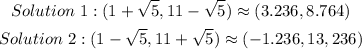
And to verify, we can graph the system:

And see that our analytic result is correct.