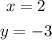Answer:
The solution to the System of equations is;
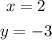
Step-by-step explanation:
Given the System of Equations;
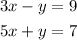
Solving by elimination.
to eliminate y, let add equation 1 and 2 together;

Since we have the value of x let us now substitute into equation 2 to get the value of y;
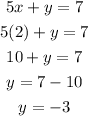
Therefore, the solution to the System of equations is;