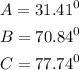Question

A little boy makes a triangular toy bin for his trucks in an inside corner of his room. The lengths of the sides of the triangular toy bin are 16 inches, 29 inches, and 30inches. What are the respective angles, in degrees? Round to the nearest hundredth.

Answer 1

Step-by-step explanation

In the question, we are given that the triangular toy bin has three sides 16 inches, 29 inches and 30 inches. To find the respective angles for each side, we will use the cosine rule.

Lets name each side with letters.

`\begin{gathered} a=16\text{ Inches} \\ b=29\text{ Inches} \\ c=30\text{ Inches} \end{gathered}`

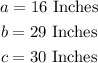
To find the angles we will use the formula below;

`\begin{gathered} Cos\text{ A=}(b^2+c^2-a^2)/(2bc) \\ CosB=(a^2+c^2-b^2)/(2ac) \\ Cos\text{ C =}(a^2+b^2-c^2)/(2ab) \end{gathered}`

We can then insert the sides to have;

`\begin{gathered} CosA=(29^2+30^2-16^2)/(2*30*29)=(99)/(116) \\ CosB=(16^2+30^2-29^2)/(2*16*30)=(21)/(64) \\ Cos\text{ C =}(16^2+29^2-30^2)/(2*16*29)=(197)/(928) \\ \end{gathered}`

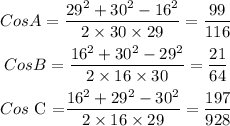
We can then derive the angle as;

`\begin{gathered} A=Cos^(-1)(1485)/(928)=31.41^0 \\ B=Cos^(-1)(21)/(64)=70.84^0 \\ C=Cos^(-1)(197)/(928)=77.74^0 \end{gathered}`

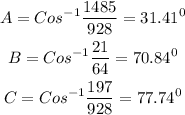
Answer:

`\begin{gathered} A=31.41^0 \\ B=70.84^0 \\ C=77.74^0 \end{gathered}`