Question

Solve this system by substitution. y = 4x - 9 y = 6x - 15 +Remember to write your answer as a coordinate point (x, y).

Answer 1

x= 3;

y= 3.

Explanation:

1. Write the equations.

`(1)y = 4x - 9 \\\\ (2)y = 6x - 15`

2. Substitute y on equation 1 by the argument of equation 2.

`(1)(6x - 15) = 4x - 9 \\\\`

3. Add 15 to both sides of the equation.

`6x - 15+15 = 4x - 9+15\\ \\6x=4x+6`

4.Subtract 4x from both sides.

`6x-4x=-4+4x+6\\ \\2x=6`

5. Divide both sides of the equation by 2.

`(2x)/(2) =(6)/(2) \\ \\x=3`

6. Find the value of y.

Now that we have a value for x, you may substitute the value of x in any of the equations in order to get a value for y. Let's plug the value into equation 2:

`y = 6(3) - 15\\ \\y=18-15\\ \\y=3`

7. Express the results.

x= 3;

y= 3.

Answer 2

We will solve as follows:

*Since in both equations is solved for y, then we equal them and solve first for x:

`4x-9=6x-15\Rightarrow2x=6\Rightarrow x=3`

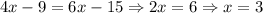
Now, we replace x in one of the equations to solve for y:

`y=4(3)-9\Rightarrow y=3`

So, the solution for the system is the point (3, 3). It can be clearly seen in the following graph: