Question

Find the angle between the vectors (-9, -7) and (8. - 4). Carry your intermediate computations to at least 4 decimal places. Round your final answer to thenearest degree

Answer 1

To find the angle between the vectors (-9, -7) and (8, - 4).

Step-by-step explanation:

Given corresponding vector points are (-9, -7) and (8,- 4).

we have that,

`\vec{a}\vec{.b}=\vec\vec|cos\theta`

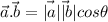
To find the angle theta.

The given vectors are,

`\begin{gathered} \vec{a}=-\vec{9i}-\vec{7j}\text{ } \\ \vec{b}=\vec{8i}-\vec{4j} \end{gathered}`

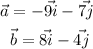
Solving for the required values we get,

`\veca|=√(81+49)=√(130)`
`\vec|=√(64+16)`

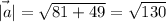
Also,