Question

Last year, Chang had $10,000 to invest. He invested some of it in an account that paid 5% simple interest per year, and he invested the rest in an account that paid 8% simple interest per year. After one year, he received a total of $740 in interest. How much did he invest in each account?Solve by tax rate or interest rate using system of linear equations.

Answer 1

From the question, we have that:

1. Chang had $10,000 to invest.

2. Let x the part Chang invested in an account that paid 5% simple interest per year.

3. Let y the part Chang invested in an account that paid 8% simple interest per year.

4. After one year, he received $740 in interest.

Then we can translate the situation as follows:

`\begin{gathered} (5)/(100)x+(8)/(100)y=740 \\ \\ x+y=10000 \end{gathered}`

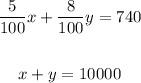
And we can solve this system using the substitution method to find x and y as follows:

6. We can multiply the first equation by 100 as follows:

`\begin{gathered} 100((5)/(100)x+(8)/(100)y)=100*740 \\ \\ 5x+8y=74000 \end{gathered}`

7. Substituting y in terms of x, we have:

`\begin{gathered} x+y=10000\Rightarrow y=10000-x \\ \\ 5x+8y=74000 \\ \\ 5x+8(10000-x)=74000 \end{gathered}`

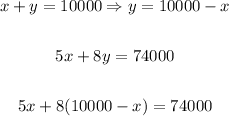
8. Using the distributive property, we have:

`\begin{gathered} 5x+80000-8x=74000 \\ 5x-8x=74000-80000 \\ -3x=-6000 \\ x=(-6000)/(-3) \\ x=2000 \end{gathered}`

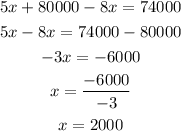
Therefore, x = $2000, and knowing that:

`\begin{gathered} x+y=10000 \\ 2000+y=10000 \\ y=10000-2000 \\ y=8000 \end{gathered}`

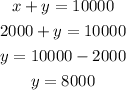
If we apply one of the above expressions, we have:

`\begin{gathered} (5)/(100)x+(8)/(100)y=740 \\ \\ (5)/(100)2000+(8)/(100)8000=740 \\ \\ 740=740 \end{gathered}`

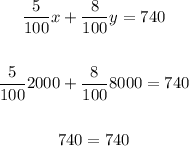
Therefore, in summary, Chang invested $2000 at 5% of simple interest, and $8000 at 8%. He invested

First account (5%) = $2000.

Second account (8%) = $8000.