Question

The height h (in feet) of a projectile shot up into the air, at time t (in seconds) is given by the formula h = −16t2 + 128t. Find the time t required for the projectile to return to its starting point

Answer 1

Given:

The height of the projectile shooting up into the air is given as,

`h\text{ = -16t}^2+128t`

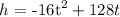
Required:

The time t required for the projectile to return to its starting position.

Step-by-step explanation:

When the projectile starts from its initial position and comes back to the same position then the height becomes zero.

Equating the given equation to zero.

`\begin{gathered} -16t^2+\text{ 128t = 0} \\ \end{gathered}`

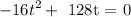
Calculating the roots of the given quadratic equation.

`\begin{gathered} 16t^2-128t\text{ = 0} \\ t(16t-128)\text{ = 0} \end{gathered}`

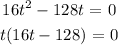
On simplifying further,

`\begin{gathered} t\text{ = 0 or 16t - 128 = 0} \\ t\text{ = 0 or t = }(128)/(16) \\ t\text{ = 0 or t = 8} \end{gathered}`

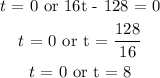
Therefore,

At t = 0 seconds, the projectile was shot into the air. After 8 seconds the projectile returned back to its original position.

Answer:

Thus the time taken by projectile to return back to its starting position is 8 seconds.