First, we need to define what a like term is:
• two or more terms are like terms if they have the same variable, or if they are independent terms (terms without variable).
For example:
2x and 8x are like terms because they both have the same variable.
3 and 12 are like terms because they both don't have variables.
With this in mind, let's answer our problem:
Problem 1:
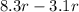
Since both terms have the same variable "r" they are like terms and we can combine them by subtracting 3.1 from 8.3, the result will be:
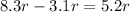
Problem 2:

In this case, the like terms are 26a and 17a because both of those terms have the same variable "a". 14 is not a like term with them because 14 does not have a variable, so we can only combine 26a and 17a by adding 26 and 17:
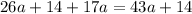
As you can see, in the result we get 43a (which is the result of the sum 26a+17a) and since 14 was not a like term, it goes as it is to the result.
Problem 3:
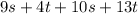
Here 9s and 10s are like terms because they have the same variable, we can combine them 9s+10s=19s.
Also, 4t and 13t are like terms, so we combine them: 4t+13t=17t.
And the result is:
