The given dataset is:
40, 39, 37, 26, 25, 40, 35, 34, 26, 39, 42, 33, 26, 25, 34, 38, 41, 34, 37, 39, 32, 30, 22, 38, 36, 28, 27, 39, 34, 26, 36, 38, 25, 39, 23, 8
The frequency = 36
The center of the data is determined by the mean (Since mean is a measure of central tendency)
Mean = Total/Frequency
Mean = 32.53
The spread of the data can be determined by the standard deviation
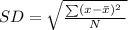
Inputing all the values of x and the mean into the formula above:
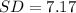
The box-and-whisker plot for the data is shown below
From the box plot shown above
Median: 34
Minimum: 8
Maximum: 42
First quartile: 26
Third quartile: 38.75
Interquartile Range: 12.75