Question

In the diagram alongside QP // YZ. QZ = 15cm and XP = 5cm. It is further given that XY is 35cm. Determine the length of: 4.1.1 PY 4.1.2 XQ

Answer 1

`\begin{gathered} PY\text{ = 30 }cm \\ XQ\text{ = 2.5 }cm \end{gathered}`

Step-by-step explanation:

Here, we want to determine the length of PY and XQ

From what we have here, there are 2 triangles, a smaller one sitting on top a larger one

When two triangles are similar, the ratio of their corresponding sides are equal

Mathematically, we have it that:

`XY\text{ = XP + PY }`

We have it that XY = 35 cm and PX is 5 cm

Thus:

`\begin{gathered} PY\text{ = XY-XP} \\ PY\text{ = 35-5 = 30 }cm \end{gathered}`

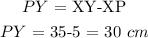
Secondly, we want to get the length of XQ as follows:

`\begin{gathered} (XP)/(XY)\text{ }=\text{ }(XQ)/(XZ) \\ \\ XZ\text{ = XQ+ 15} \\ \text{Let us call XQ x} \\ We\text{ have it that:} \\ (5)/(35)\text{ = }(x)/(x+15) \\ 5(x+15)\text{ = 35(x)} \\ 5x+75\text{ = 35x} \\ 75\text{ = 35x-5x} \\ 30x\text{ = 75} \\ x=\text{ }(75)/(30) \\ x=\text{ }(5)/(2) \\ x\text{ = 2.5 }cm\text{ } \\ XQ\text{ = 2.5 }cm \end{gathered}`