Question

3.Write an equation to represent each situation. Then solve the system ofequations to answer the question.Jake pays a monthly rate of $42 for cell service and$0.28 for each minute over 300 minutes.Carlos pays a flat rate of $80 per month forunlimited minutes.For how many minutes in one month will Jake andCarlos pay the same amount?

Answer 1

First, let's write the equation for Jake. We know that Jake pays a monthly rate of $42, let's say that the cost is "y", and "x" is how many minutes Jake used (over 300), therefore, Jake will pay

`y=42+0.28x`

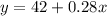
$42 plus $0.28 for each "x" minute that he exceeds over 300. Just to make sure, the equation for Jake is

`y=42+0.28x`

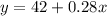
ATTENTION! "x" is not how many minutes Jake used it, it's how many minutes Jake talked over 300.

The equation for Carlos will be very simple, it's just $80 per month, always, then the cost "y" will be

`y=80`

As we can see, now we have a system of equations

`\begin{cases}y=42{+0.28}x \\ y={80}\end{cases}`

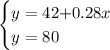
It's a very simple system to solve because we already have the value of y, it's 80, therefore

`\begin{gathered} 80=42+0.28x \\ \\ 0.28x=80-42 \\ \\ 0.28x=38 \\ \\ x=(38)/(0.28)=135.714286 \end{gathered}`

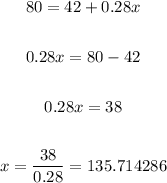
Therefore, if Jake approximately exceeds 135.71 minutes, he will pay the same as Carlos, but, the question asks for how many minutes in one month! then we must add 300 to the result because we must include the minutes that Jake doesn't pay (under 300)

`\text{ minutes = 435.71}`

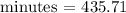
The final result is

`\text{m}\imaginaryI\text{nutes=435.71}`

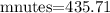
For 435.71 minutes in one month, Jake and Carlos will pay the same amount.