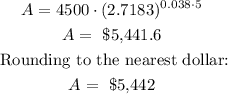Answer:
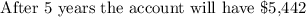
Explanation:
Continuously compounded interest means that an account balance is constantly earning interest, as well as refeeding that interest back into the balance so that it, too, earns interest. It is represented as:
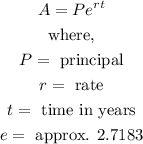
Then, for $4,500 invested at a rate of 3.8%, after 5 years you will have: