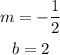Question

Please help if you can.Can you do 4 b) and c)

Answer 1

The equation of a line in its slope-intercept form is

`\begin{gathered} y=mx+b \\ \text{ Where m is the slope of the line and } \\ b\text{ is the y-intercept} \end{gathered}`

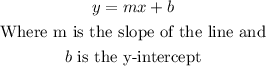
Then, to find the slope and the y-intercept of the given lines, you just have to take the equations to their slope-intercept form.

For point 4 b) you have

`\begin{gathered} 5x-10y=20 \\ \text{ Subtract 5x from both sides of the equation} \\ 5x-10y-5x=20-5x \\ -10y=20-5x \\ \text{ Divide by -10 into both sides of the equation} \\ (-10y)/(-10)=(20-5x)/(-10) \\ y=-(20)/(10)-(5x)/(-10) \\ y=-2+(1)/(2)x \\ \text{ Reordering} \\ y=(1)/(2)x-2 \end{gathered}`

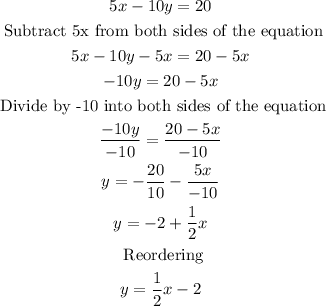
Therefore, the slope and the y-intercept of this equation are

`\begin{gathered} m=(1)/(2) \\ b=-2 \end{gathered}`

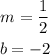
For point 4 c) you have

`\begin{gathered} x+2y=4 \\ \text{ Subtract x from both sides of the equation} \\ x+2y-x=4-x \\ 2y=4-x \\ \text{ Divide by 2 into both sides of the equation} \\ (2y)/(2)=(4-x)/(2) \\ y=(4)/(2)-(x)/(2) \\ y=2-(1)/(2)x \\ \text{ Reordering} \\ y=-(1)/(2)x+2 \end{gathered}`

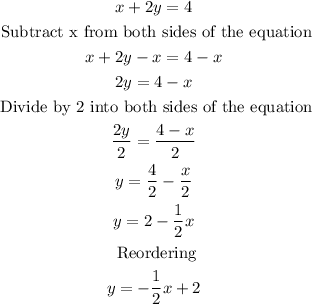
Therefore, the slope and the y-intercept of this equation are

`\begin{gathered} m=-(1)/(2) \\ b=2 \end{gathered}`