Recall that the general form of a straight line is
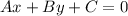
where A,B and C are real numbers.
To find this equation, we will first find the slope intercept form of the line and then apply mathematical operations so we get the form we are looking form. Recall that the slope-intercept form of a line is of the form

where m is the slope and b is the y intercept. We will first find the slope.
Recall that the slope of a line that passes through the points (a,b) and (c,d) is given by the formula
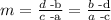
so in our case we have a=5,b=2, c=-1 and d=4. So we get
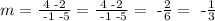
So far, our line equation would look like this
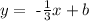
Note that as we want this line to pass through the point (5,2), this means that if we replace x=5 in this expression we should get y=2. So we have
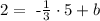
so if we add 5/3 on both sides, we get
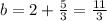
so our equation becomes
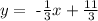
Now, from this equation we can look for the general form. First, we will multiply both sides by 3, so we get
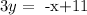
now, we add x on both sides, so we get
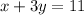
Finally, we subtract 11 on both sides, so we get
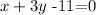
which is the general form of the line