Question

A flexible container has a volume of 14.5 L under a pressure of 135 kPa and a temperature of 15.3 C if the temperature is increased to 58.5 C and the volume is increased to 27.6 L what is the pressure un atmospheres?

Answer 1

The final pressure of the gas is 81.54kPa

Step-by-step explanation

Given that;

0. The initial volume of the gas is 14.5L

,

1. The initial temperature of the gas is 135 degrees celcius

,

2. The initial pressure of the gas is 135kPa

,

3. The final temperature of the gas is 58.5 degrees celcius

,

4. The final volume of the gas is 27.6L

Follow the steps below to find the final pressure of the gas

Step 1; Write the general gas law equation

`\text{ }\frac{\text{ P1V1}}{\text{ T1}}\text{ }=\text{ }\frac{\text{ P2V2}}{\text{ T2}}`

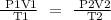
Step 2; Convert the temperature to degrees Kelvin

`\begin{gathered} \text{ T K = t}\degree C\text{ + 273.15} \\ \text{ for t1 = 15.3}\degree C \\ \text{ T K = 15.3 + 273.15} \\ \text{ T K = 288.45K} \\ \\ \text{ For t2 = 58.5}\degree C \\ \text{ T K = 58.5 + 273.15} \\ \text{ T K = 331.65K} \end{gathered}`

Step 3; Substitute the given data into the formula in step 1 to find the final pressure of the gas

`\begin{gathered} \text{ }\frac{\text{ P1V1}}{\text{ T1}}\text{ }=\text{ }\frac{\text{ P2V2}}{\text{ T2}} \\ \\ \text{ }\frac{\text{ 135}*\text{ 14.5}}{288.45}\text{ }=\text{ }\frac{\text{ P2}*\text{ 27.6}}{331.65} \\ \text{ Cross multiply} \\ \text{ 135}*\text{ 14.5 }*\text{ 331.65 }=\text{ P2 }*\text{ 27.6}*\text{ 288.45} \\ 649204.875\text{ = 7961.22 P2} \\ \text{ Divide both sides by 7961.22} \\ \text{ }\frac{\text{ 649204.875}}{7961.22}\text{ }=\text{ }\frac{\cancel{7961.22}P2}{\cancel{7961.22}} \\ \text{ P2 = 81.54kPA} \end{gathered}`

Therefore, the final pressure of the gas is 81.54kPa