SOLUTION
Step 1 :
In this question, we are given that the height ( in feet ) of a ball thrown by a child is :
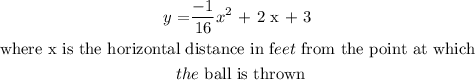
Step 2 :
a) How high is the ball when it leaves the child's hand?
Answer:
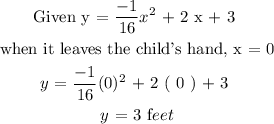
b) What is the maximum height of the ball?
Answer:

c) How far from the child does the ball strike the ground?
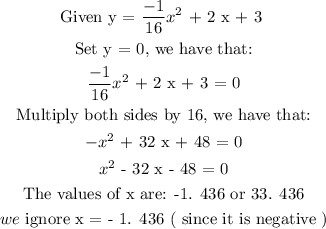
But the child threw the ball from x = 0,
It means that the ball landed 33. 436 feet away from the child.