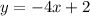We have the following equation:

In order to locate the slope of this line equation, lets isolate the variable y. Then, by subtracting 2x and 20 to both sides, we have
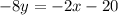
and by dividing both sides by -8, we obtain
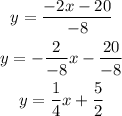
By comparing this result with the slope-intercept form

we can see that the slope m of the given line equation is
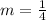
Since we need a perperdicular line, we need to compute the negative reciprocal of the slope m, that is,
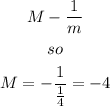
Then, the perpendicular line has the form
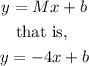
Finally, we can find the y-intercept b by substituting the coordinates of the given point (-1,6). It yields,
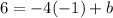
which gives
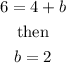
Therefore, the perpendicular line to 2x-8y+20=0 that goes throught the point (-1,6) is: