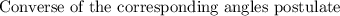10. Using the converse of the alternate interior angle theorem, we can prove that line J is parallel to line K.
Given:

Prove: J || K
Statement:

Reason: Given
Statement:

Reason: Vertical angles theorem
Statement:

Reason: Transitive property of congruence
Statement:
J || K
Reason: