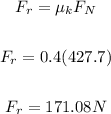Answer:
1) Free Body Diagram is drawn below
2) Newton's first law of motion is stated below
3) Force, F = 182.2 N
Normal Force = 427.7 N
Frictional force = 171.08 N
Step-by-step explanation:
The Free Body diagram is drawn below:
Newton's first law of motion states that a body will remain in a state of rest or uniform motion unless it is acted upon by an external source
The magnitude of the force F is calculated below
Since the object is at constant velocity, the acceleration, a = 0 m/s^2
![\begin{gathered} \sum F=ma \\ \\ Fcos20-F_r=50(0) \\ \\ Fcos20-F_k=0.........................* \\ \\ Note: \\ F_k=\mu(mg-Fsin20) \\ \\ F_k=0.4[(50*9.8)-0.34F] \\ \\ F_k=196-0.136F..................** \\ \\ Substitute\text{ \lparen **\rparen into \lparen *\rparen} \\ \\ Fcos20-(196-0.136F)=0 \\ \\ 0.94F-196+0.136F=0 \\ \\ 1.076F=196 \\ \\ F=(196)/(1.076) \\ \\ F=182.2N \\ \end{gathered}](https://img.qammunity.org/2023/formulas/physics/college/limns1alrxuqsx78fe7efi7tp88wmoe6fk.png)
The normal force is calculated below
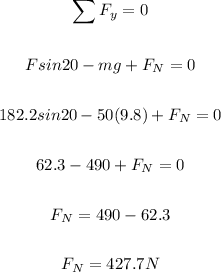
The frictional force is calculated below