The domain of a function is all values of x the function can assume.
In this function, x can assume any real value but zero (because there would be a fraction with zero in the denominator), so the domain is:
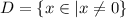
The range of a function is all values of f(x) the function can assume.
In this function, f(x) can assume any positive number (greater than zero), so the range is:
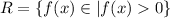
In order to find the inverse function, we just need to switch x by f^-1(x) and f(x) by x, and then isolate f^-1(x), so we have:
![\begin{gathered} f(x)=(16)/(x^4) \\ x=(16)/((f^(-1)(x))^4) \\ (f^(-1)(x))^4=(16)/(x) \\ f^(-1)(x)=\sqrt[4]{(16)/(x)} \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/yszqh2j3buv9af1lt8gcv8lv1il9n8fhob.png)
f(x) is a function, since any value of x has only one corresponding value of f(x).