Question

a.Calculate SSx, SSy, and SSxy.b. Calculate r. Is there sufficient evidence to support that the linear correlation betweenx and y exists (using a = 0.05)?c. If the linear correlation exists, construct the least squared line.d. If the linear correlation exists, draw the scatter plot with the line. Otherwise, draw thescatter plot.

Answer 1

Given the values in the table, the following are the solutions to the questions.

Question A: SSx, SSy and SSxy

To get SSx:

`\begin{gathered} SS_x=\sum ^{}_{}(x-\bar{x})^2=\sum ^{}_{}x^2-\frac{(\sum^{}_{}x)^2}{n} \\ \sum ^{}_{}x^2=1+4+9+16+25=55_{} \\ (\sum ^{}_{}x)^2=(1+2+3+4+5)^2=225_{} \\ n=5 \\ SS_x=\sum ^{}_{}x^2-\frac{(\sum^{}_{}x)^2}{n}=55-(225)/(5) \\ =55-45=10 \end{gathered}`

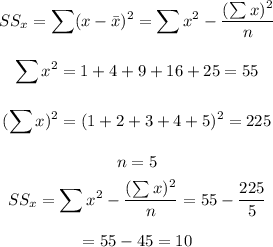
To get SSy:

`\begin{gathered} SS_y=\sum ^{}_{}(y-\bar{y})^2=\sum ^{}_{}y^2-\frac{(\sum ^{}_{}y)^2}{n} \\ (\sum ^{}_{}y)^2=(14+11+9+9+6)^2=49^2=2401 \\ \sum ^{}_{}y^2=(196+121+81+81+36)=515 \\ n=5 \\ SS_y=515-(2401)/(5)=515-480.2=34.8 \end{gathered}`

To get SSxy:

`\begin{gathered} SS_(xy)=\sum ^{}_{}(x-\bar{x})(y-\bar{y})=\sum ^{}_{}xy-\frac{(\sum ^{}_{}x)(\sum ^{}_{}y)}{n} \\ \sum ^{}_{}xy=(14+22+27+36+30)=129 \\ \sum ^{}_{}x=15 \\ \sum ^{}_{}y=49 \\ SS_(xy)=129-((15)(49))/(5)=129-147=-18 \end{gathered}`

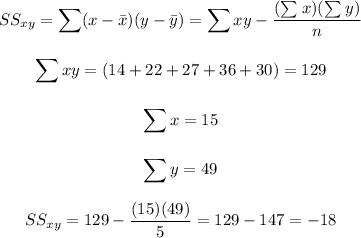
Question b: Calculate r

`\begin{gathered} r=\frac{SS_(xy)}{\sqrt[]{SS_x.SS_y}} \\ r=-\frac{18}{\sqrt[]{(10)(34.8)}}=\frac{-18}{\sqrt[]{348}}=-0.9649 \end{gathered}`

Given the value gotten above, I will need to calculate the critical value using the critical value table to ascertain if there is enough evidence.

`\alpha=0.05,degree\text{ of fre}edom=n-2=5-2=3`

Using the critical value table to get the value at significance level 0.05 and degree of freedom 3, the gotten value gives 0.878 i.e, 0.878 or -0.878. Since the value of r of -0.9649 falls within range of -0.878, we reject the null hypothesis. Hence, there is sufficient evidence to support that the linear correlation between x and y exists.

Question c: Yes, the linear correlation exists between x and y. We then construct the least squared line.

Therefore, the least squared line will be:

`\begin{gathered} y^(\prime)=a+b\bar{x} \\ y^(\prime)=18.8+(-1.8)x \\ y^(\prime)=18.8-1.8x \end{gathered}`

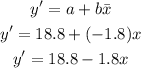
Question d:

The Scattered plot with the line will be: