47 cm and 39 cm
Step-by-step explanation
the theorem states that the sum of any two sides of a triangle is greater than or equal to the third side; in symbols, a + b > c
so
Step 1
Let
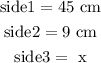
hence
a)
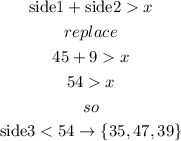
Step 2
b)

Step 3

hence
the solutions must fit:
![\begin{gathered} \text{side}3<54\rightarrow\lbrace35,47,39\rbrace \\ x>-36\rightarrow all\text{ the options}\lbrace35,58,47,54,39\rbrace \\ x>36 \\ 36therefore, the solution is the intersection of those sets[tex]\lbrace47cm,39cm\rbrace]()
47 cm and 39 cm
I hope this helps you