In order to find the inverse of f we need to take its equation and replace f(x) and x with x and f^-1(x). Then we have:
![\begin{gathered} f(x)=-4\sqrt[]{x}-1 \\ x=-4\sqrt[]{f^(-1)(x)}-1 \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/3u0b3jrzv4xoik9r8xafgyqq8w53qr3se2.png)
And we find the inverse function:
![\begin{gathered} x=-4\sqrt[]{f^(-1)(x)}-1 \\ x+1=-4\sqrt[]{f^(-1)(x)} \\ -((x+1))/(4)=\sqrt[]{f^(-1)\mleft(x\mright)} \\ f^(-1)(x)=((x+1)^2)/(16) \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/ilzsgvg39uo29h4osh6alniufl7lnkxays.png)
So this is the inverse function but we still have to find the inequality for if it has one. First is important to remember that the x in the last calculation replaced f(x). This means that the inequalities that f(x) meets are the same that x meets. So let's see, we have:
![f(x)=-4\sqrt[]{x}-1](https://img.qammunity.org/2023/formulas/mathematics/college/m8yojw7sq56cwbkpdy389e2xtu4wm1ibtd.png)
The square root of x can have as a result any number between 0 and infinite. This means that f(x) tends to negative infinite (when the square root tends to infinite) and that the maximum value of f(x) is:
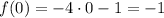
This means that:

And since we replace f(x) by x then for the inverse function we have:
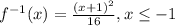
Then the answer is the fourth option.