ANSWER
-18.11
Step-by-step explanation
We want to find the mean of the probability distribution.
To do that, we apply the formula:
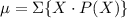
This means that we have to find the product of each value has its corresonding probability and find the sum:
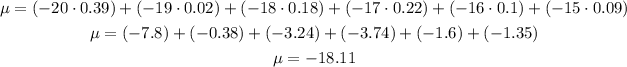
That is the mean of the probability distribution.